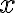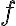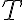Browse the glossary using this index

Special | A | B | C | D | E | F | G | H | I | J | K | L | M | N | O | P | Q | R | S | T | U | V | W | X | Y | Z | ALL

I

Invariance and equivariance

by Yee Wei Law - Monday, 6 January 2025, 3:50 PM

A function $f(x)$ of an input $x$ is invariant to a transformation $T(x)$ if

$f(T(x)) = f(x).$

In other words, function $f$ in invariant to transformation $T$ if produces the same output regardless of the output of $T$ ^{[Pri23, §10.1]}.

For example, an image classifier should be invariant to geometric transformations of an image.

A function $f(x)$ of an input $x$ is equivariant or covariant to a transformation $T(x)$ if

$f(T(x)) = T(f(x)).$

In other words, function $f$ is equivariant or covariant to transformation $T$ if the output of $f$ changes in the same way under $T$ as the input^{[Pri23, §10.1]}.

For example, when an input image is geometrically transformed in some way, the output of an image segmentation algorithm should be transformed in the same way.

References

[Pri23]

S. J. Prince, Understanding Deep Learning, MIT Press, 2023. Available at http://udlbook.com.

Keyword(s):

Cyber Engineering Knowledge Base

Artificial intelligence (including machine learning which includes deep learning)

I

Invariance and equivariance

References